How To Calculate Confidence Interval

December 10, 2023 admin 0 Comments Calculate Confidence Interval, How To Calculate Confidence Interval

I. Introduction to Confidence Intervals

In the realm of statistics, confidence intervals are a crucial tool used to estimate the range within which a population parameter lies. These intervals provide a level of certainty about the true value of a statistic derived from a sample.

II. Understanding the Concept of Confidence Intervals

A. Definition and Purpose

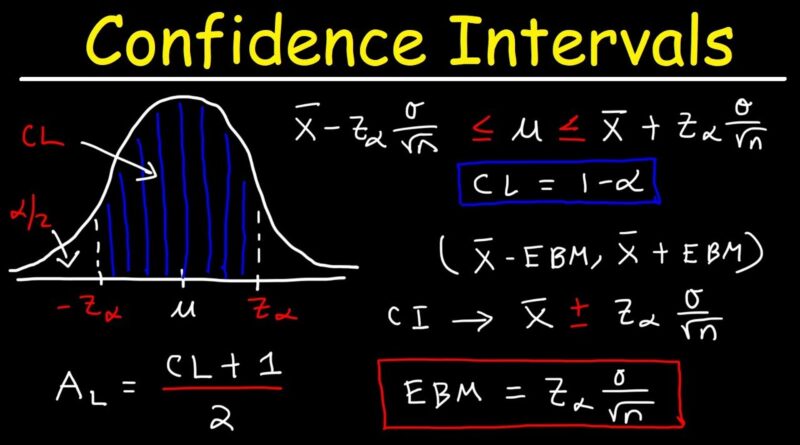
A confidence interval is a range of values, derived from sample statistics, that is believed to contain the true population parameter with a specified probability. It serves the purpose of gauging the reliability of estimates derived from sample data.

B. Importance in Statistics

Confidence intervals offer a way to assess the precision of an estimate and help researchers make informed decisions based on data analysis. They play a pivotal role in hypothesis testing and determining the significance of results.

III. Formula and Calculation Process

A. Basic Formula

The formula for calculating a confidence interval involves the sample mean, standard deviation, sample size, and a critical value based on the desired confidence level.

B. Step-by-Step Calculation

The process includes determining the mean and standard deviation, selecting the confidence level, finding the critical value from statistical tables or software, and applying the formula to compute the interval.

IV. Factors Affecting Confidence Intervals

A. Sample Size

A larger sample size generally results in narrower intervals, increasing the precision of estimates.

B. Confidence Level

Different confidence levels, such as 90%, 95%, or 99%, impact the width of the interval, affecting the level of certainty.

C. Variability in Data

Higher variability in the data widens the confidence interval, indicating less precision in the estimate.

V. Interpretation of Confidence Intervals

A. Statistical Significance

Confidence intervals aid in determining whether an observed effect is statistically significant, providing insights into the significance of results.

B. Practical Significance

Beyond statistical significance, these intervals assist in understanding the practical implications of the findings in real-world scenarios.

VI. Applications and Examples

A. Real-life Scenarios

From medical research to business forecasts, confidence intervals are utilized to make informed decisions in various fields.

B. Practical Applications

They are applied in market research, quality control, and policy-making to assess the reliability of data-driven conclusions.

VII. Common Mistakes to Avoid

A. Misinterpreting Results

Incorrectly interpreting the confidence interval can lead to erroneous conclusions about the population parameter.

B. Incorrect Sampling Methods

Improper sampling techniques can skew results and compromise the accuracy of confidence intervals.

VIII. Enhancing Accuracy in Calculations

A. Increasing Sample Size

A larger sample size enhances the precision of estimates, narrowing the confidence interval.

B. Adjusting Confidence Levels

Choosing different confidence levels can alter the width of intervals, impacting the certainty of estimates.

IX. Concluion

In conclusion, mastering the calculation of confidence intervals is pivotal in statistical analysis. These intervals serve as invaluable tools in assessing the reliability of data and drawing meaningful conclusions.

Technoowrites